The Interval Pattern Of Fourths: The NANDI Method
Check out The NANDI Method on YouTube! Below is our companion video to this lesson!
It’s fairly easy to visualize the twelve scale degrees as you move from the root to the octave using half-steps. Look at the diagram below and you will see all twelve of the scale degrees in the key of C, played on the second (B) string:

Pretty simple, right?
It’s a single sequence of notes that are all played on one string. Of course, you will use more than one string on the guitar when you are playing it. This is why you need to understand how all twelve scale degrees are played across all six of the strings.
Take a look at the diagram below:
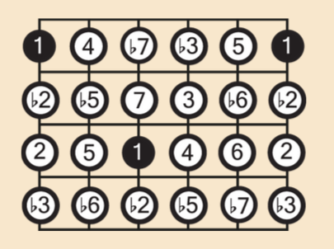
A bit confusing, right?
Luckily, it becomes much easier to understand once you apply the NANDI (Note After Note Decoding Intervals) system. We say that this system helps guitarists to decode the fretboard, as it truly helps you to figure out the relationships of notes and intervals without having to solely rely on memorization techniques.
When you study the guitar, you realize that the primary interval found on it is the fourth. When you reorder the twelve interval numbers in a scale in a pattern of fourths, you get this pattern:
1, 4, ♭7, ♭3, ♭6, ♭2, ♭5, 7, 3, 6, 2, 5, 1
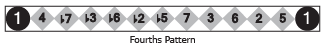
To make it even easier to understand, let’s break the pattern up into two unique sections. The 7-3-6-2-5 sequence appears twice, once with all flats (♭7-♭3-♭6-♭2-♭5) and once with all natural intervals (7-3-6-2-5). Remember, the flat interval number sequence is preceded by 1 and 4, while the natural interval number sequence is followed by 1 and 4.
![]()
Let’s begin by looking at the natural notes, which we can assume we’re in the key of C when we look at it. If we only want to see all of the natural notes in the fourth pattern, the sequence looks like this:
![]()
Of course, you are probably not going to start your scale off on the seventh degree of the scale. In fact, you’ll likely start out using the root or the 1. There are a few ways that we can locate the fourth starting with the root.
One of the simplest ways is to simply count up five chromatic intervals starting from the root 1 (♭2, 2, ♭3, 3, 4).
The fastest way of doing this might be the most useful if you are having trouble remembering the sequence of fourths in the interval pattern. You can figure it out by subtracting a whole-step from any two intervals that are found a perfect fourth apart.
![]()
One of the easiest fourth intervals to remember is the one from 1 to 4. If you move a whole-step down from 1 and 4, you get ♭7 and ♭3. Whole-step down from ♭7 and ♭3 is ♭6 and ♭2. A whole-step down from ♭6 and ♭2 is ♭5 and 7, a whole-step down from 7 and 3 is 6 and 2, and finally, a whole-step down from 6 and 2 is 5 and 1.
Applying This To The Fretboard
Now that you have a better idea of the sequence, let’s see how we can apply it to the fretboard. The mnemonic device that we use to apply sequences to our fretboard is:
One Forsaken Tree Set To Fire
This can help us to remember the scale degrees, “One, Four, Seven, Three, Six, Two, Five” We can use this mnemonic device to move across the fretboard in fourths:

To maintain our pattern of fourths, we must move up one fret when we get to our second B string. Look at the pattern above to see what happens when we begin our sequence on a root, though note that this will lead us into flat notes, which are outside the major scale.
If we want to play only the natural intervals in the major scale (1, 2, 3, 4, 5, 6, 7), then we must arrange them in an order using fourths. This will give us 7,3,6,2,5,1.
We can use the following mnemonic device to remember this pattern:
Severed Tree Sticks To Fight On.
When you play across the fretboard, and start on the seventh which is one fret below the root, it will look a little something like this: